Almost all the biggest math enthusiasts I’ve known have also loved classical music, especially the work of Bach, Mozart, and Beethoven. Of course, as San Francisco Symphony music director Michael Tilson Thomas once put it, you can’t have those three as your favorite composers, because “they simply define what music is.” But don’t tell that to the mathematically minded, on whom all of them, especially Bach and Beethoven, have always exerted a strong pull.
But why? Do their musical compositions have some underlying quantitative appeal? And by the way, “how is it that Beethoven, who is celebrated as one of the most significant composers of all time, wrote many of his most beloved songs while going deaf?” The question comes from a TED-Ed segment and its accompanying blog post by Natalya St. Clair which explains, using the example of the “Moonlight Sonata,” what the formidable composer did it using math. (You might also want to see St. Clair’s other vides: The Unexpected Math Behind Van Gogh’s “Starry Night.”)
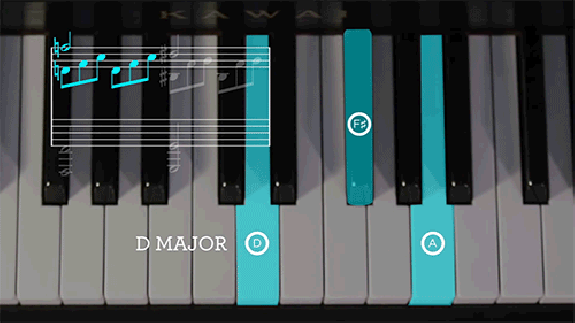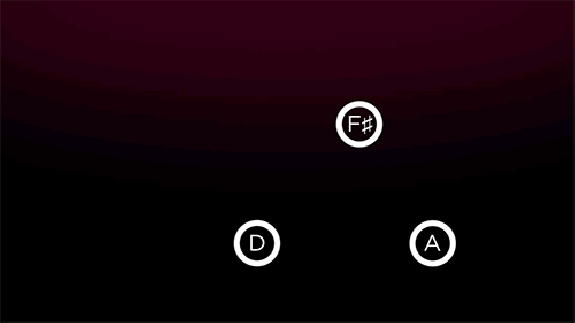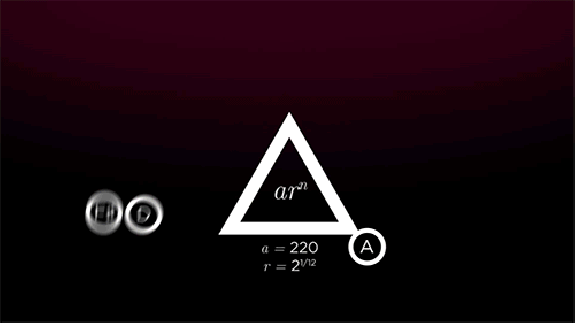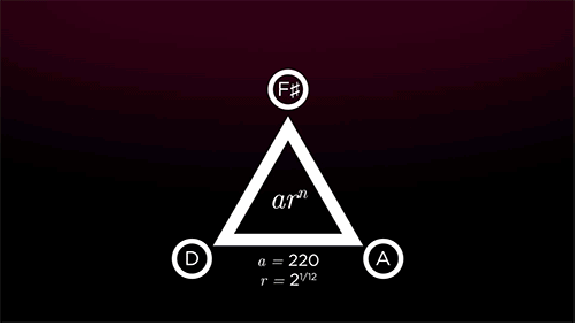
“The standard piano octave consists of 13 keys, each separated by a half step,” St. Clair writes. “A standard major or minor scale uses 8 of these keys with 5 whole step intervals and 2 half step ones.” So far, so good. “The first half of measure 50 of ‘Moonlight Sonata’ consists of three notes in D major, separated by intervals called thirds that skip over the next note in the scale. By stacking the first, third, and fifth notes — D, F sharp, and A — we get a harmonic pattern known as a triad.” These three frequencies together create “ ‘consonance,’ which sounds naturally pleasant to our ears. Examining Beethoven’s use of both consonance and dissonance can help us begin to understand how he added the unquantifiable elements of emotion and creativity to the certainty of mathematics.”
Explained in words, Beethoven’s use of mathematics in his music may or may not seem easy to understand. But it all gets clearer and much more vivid when you watch the TED-Ed video about it, which brings together visuals of the piano keyboard, the musical score, and even the relevant geometric diagrams and sine waves. Nor does it miss the opportunity to use music itself, breaking it down into its constituent sounds and building it back up again into the “Moonlight Sonata” we know and love — and can now, having learned a little more about what mathematician James Sylvester called the “music of the reason” underlying the “mathematics of the sense,” appreciate a little more deeply.
Related Content:
Stream the Complete Works of Bach & Beethoven: 250 Free Hours of Music
Beethoven’s 5th: The Animated Score
Leonard Bernstein Conducts Beethoven’s 9th in a Classic 1979 Performance
Beethoven’s Ode to Joy Played With 167 Theremins Placed Inside Matryoshka Dolls in Japan
Man Hauls a Piano Up a Mountain in Thailand and Plays Beethoven for Injured Elephants
Slavoj Žižek Examines the Perverse Ideology of Beethoven’s Ode to Joy
Oliver Sacks’ Last Tweet Shows Beethoven’s “Ode to Joy” Movingly Flashmobbed in Spain
Based in Seoul, Colin Marshall writes and broadcasts on cities, language, and style. He’s at work on a book about Los Angeles, A Los Angeles Primer, the video series The City in Cinema, the crowdfunded journalism project Where Is the City of the Future?, and the Los Angeles Review of Books’ Korea Blog. Follow him on Twitter at @colinmarshall or on Facebook.


That was a terrible video that explained nothing of the genius of Beethoven and only loosely explained harmonic principles that apply to EVERY composer. Also, an octave consists of 12 chromatic notes and major/minor scales use 7 notes. The outer notes they use in the C–C examples are the same.
Physics teachers and physics researchers befuddle their students with the cliché: “Some state are forbidden.” Or words to that effect. As Sir James Jeans explains in the pages of ‘Science and Music’ (1937) https://ia800504.us.archive.org/27/items/sciencemusic00jean/sciencemusic00jean.pdf Pages: 65–69
Vibrating strings, strings in resonance can only assume whole number multiples of their fundamental frequency.
And so it is with atoms and molecules. A fact of nature unknown to every physics professor in the world. Jean’s explanation could be a part of every high school and college physics text book. It could be. …
Many thanks for this post and its links.
Hugely informative.
To commenter @Alan Drabke: thankyou so much for the link to the “Science and Music” by Sir James Jeans — is a real bonus.
I explain how the beginning of LVB’s 5th symphony, when translated into math, reads like the first chapter of a book on group theory; the symphony was written before mathematicians woke up to the concept of group theory; see section (67) of my book: http://www.pianopractice.org/
The Moonlight is an invention by LVB in which he superposed harmony with dissonance to bring out the beauty of harmony, and the pain of dissonance, section (49)
The Appassionata is a piano version of his 5th symphony, section (51)
The first composer to realize that musical consonance derives from shared overtone frequencies was Jean-Philippe Rameau, in his “Treatise on Harmony” (Traité de l’Harmonie).
http://www.amazon.com/Treatise-Harmony-Dover-Books-Music/dp/0486224619/
Jeans is good but not perfect. His statement that different keys cannot possibly have different tonal characteristics, for example, clearly does not apply to those of us with absolute pitch.
Another (older) book, which is heavy going at times because of all the math, is Helmholtz’s classic: “On the sensations of tone”.
http://www.amazon.com/Sensations-Tone-Dover-Books-Music/dp/0486607534/
Physics (and, for that matter, applied math) and music have been intertwined for a long time. In fact, the first person in the West to actually apply a version of equal temperament in instrument building was the lute maker Vincenzo Galilei — the father of Galileo!
This is a common mistake of — for want of a better word — a type of “presentism”. Only instead of judging history by modern standards, people make the mistake of thinking composers build music mathematically.
The mistake comes from how their music can be deconstructed mathematically — which can be done with most everything human. The reason it’s done so frequently with music is because of our love for music and how simple the language actually is: the love of music provides the motivation and the simplicity makes the deconstruction logical, neat and tidy.
But it’s still a mistake. I’ve never used any math, ever, while writing a piece. Nor have I ever known someone who did although there are a few ‘perfomance art’ composers who have done it on purpose. That work however, tends to be unlistenable from a purely enjoyment standpoint.
And being deaf wasn’t the handicap non-composers think it is. It was a challenge but mostly for reasons the average person wouldn’t think of: tempos and orchestration. Most composers can write without using an instrument — they’re experts on the subject, after all: that’s why they’re composers — and they know the notes they write will sound how they intended them to, but dialing in the correct tempos and timbres can be a challenge.
It’s because when you’re writing in your head, you’re using a perfect orchestra and you understand the emotions you’re attempting to convay and where in the score you’re attempting to convey them. So the tempo is irrelevant to you: it works in your head perfectly, at any tempo.
Think of a novelist who’s trying to explain a story they already know backwards and forwards in their head. Have you ever read a book where you feel lost at some point? The author wasn’t. He or she just forgot to give you enough information to keep you informed of what they were thinking.
And timbre is very much like tempo: much easier to mess up than the actual notes.
That’s not an attempt to dismiss the brilliant accomplishements of Beethoven’s work but merely an attempt to put it in perspective.